Intro to Mechanics

Over the last 30 years I have taught (or attempted to teach!) Mechanics to a variety of years in Mechanical Engineering degree courses, from Foundation year to Masters level. It has successively disappointed me, and subsequently appalled me, as to the level of awareness of Mechanics in students completeing their A Level education in the UK. The restrictions of the time devoted to Mechanics in the A Level syllabus, and the ability of students to avoid Mechanics and Calculus within the A Level syllabus has now reached frightening proportions. How can anyone study Mechanical Engineering,for which Mechanics is the obvious core subject, without a reasonable preparation at School? However, this is the situation we find ourselves in. The statistics are incredible, of the students taking A Level Mathematics and Physics 70% are taught by teachers who have not graduated in the relevant subjects. Furthermore, of that 70%, a further 70% have no degree in STEM subjects. So overall about 50% of A level students are taught by completely unqualified teachers. Contrast this with the position in Scotland where teachers are required to by Law to have a relevant qualification to teach a given subject!!!

We are therefore in England faced with a situation that our A Level students are, from the State Sector (this is not true of some of those students from the Private Sector), largely completely unequipped for degree study in any branch of Engineering or Physical Science that requires advanced mathematics (Calculus) and Mechanics. In my darker moments this leads me to the conclusion that we sould not ask for Maths and Physics at A Level to take Engineering, but that we should take intelligent students (however that is defined!) and teach them advanced mathematics and physics from scratch at University - at least most of the staff teaching them will have a grasp of the subjects!

This is completely anectdotal, but nonetheless true, on a recent Accreditaion vist for the IMechE, whilst talking to students and asking the question "what did you find good about the course" a Foundation Year student answered "Mathematics". Somewhat surprised by this, I asked what he meant - the response was very illuminating. He said the his teacher did not understand about the last 20% of the GCSE syllabus, and she could not cope with the A Level syllabus at all. He considered that his C grade at A level was quite good as he had had to teach himself - I concurred! His resonse was conditioned by the fact that at University, being taught Mathematics by someone who understood the subject, he found it quite simple!!!!

I have tried to embody this in what I teach, and in particular to try to embed in students a FUNDAMENTAL understanding of Mechanics. Whether you consider that so, or not, in contained in the following pages.

This website is under development, and additional pages will be added in future - inforned comments are always welcome. Use the link below to go to web pages containing pdf's of notes etc.

STATACT

Introduction to the Methodology Mechanics

Many years ago when I was a PhD student, being short of cash (as anything changed?), I undertook to metricate Schaum’s Strength of Materials, authored by William Nash. The Schaum’s series are tutorial books and contain hundreds of solved examples. Metricating a book such as this is no small task, as the problems all have to be reworked with sensible (integer) values of variables. Also there were some 750 problems for which no solutions existed, and so they had to be worked out from scratch. In all I had to do 1750 mechanics problems in a year, i.e. about 35 per week. There is no better training in mechanics if you can afford the time!!!

In going through these problems it eventually dawned upon me that I was using a standard process to solve the problems. This process is actually implicit in the original text, and is also there in most mechanics textbooks, although again hidden and never explicitly stated. I therefore compiled the method I was using and called it a ""methodology, which is shown below.

The importance of the methodology is that it is a way of thinking about problem solving at a Meta (Above) level; it is about thinking how to think. This to me is the very essence of education. Students shoul be taught at the Meta level – how to obtain methodologies to solve whole classes of problems - rather than endless specific examples in the hope that some understanding may emerge. This latter type of teaching is what I experienced, and what most students still experience.

I have used this methodology for many years, at all levels from Foundation Year to the Masters year, and all the student groups exposed to it are highly appreciative. It gives them a clear framework within which they can apply the methodology to every problem, and they feel ‘safe’. When I have introduced the methodology to students who have been taught by someone else in earlier years, they frequently ask the question “why weren’t we taught this at the start?”. This is the impetus to put the methodology on my web page.

Mechanics

- Science (study) of MOTION of objects

- Motion needs ENERGY

Step 1: Choose axis system (Generally but not restrictively 2D)

- Rectangular Co-ordinates (Cartesian)

- Normal &Tangential (N&T) Co-ordinates

STEP 2: Overall Equilibrium

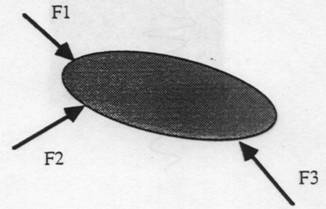
ΣFv = ΣFh = ΣM = 0

STEP 3: Method of Sections / Free Body Analysis

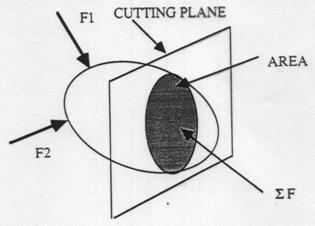
Free Body Equilibrium:

ΣFv = ΣFh = ΣM = 0              STATIC       

ΣFv = ΣFh = ΣM not = 0        DYNAMIC

= MASS x ACCn

- “Equations of Motion”

- “Degrees of Freedom”

STEP 4: Determinate or Indeterminate??

Three equations of equilibrium

< 3 unknowns - determinate

> 3 unknowns - indeterminate

STEP 5: Indeterminate Systems

Need more Independent equations – COMPATIBILITY

For a structure to move in a Compatible manner the movement of adjacent parts should be the same.

NOTE: This “Methodology” or “Paradigm” will specify all mechanics problems. Non-linear problems require a further Linearisation step and a Minimum Energy criterion.

The "Free Bodies" are FINITE therefore when combined with Matrix Methods and solved on a computer this methodology is known as FINITE ELEMENT ANALYSIS

What is Mechanics?

Mechanics is the study motion and motion needs energy. For the purposes this module it can be taken to mean the interaction between forces and bodies. It allows answers to be given to questions like:

• What set of forces can be applied to a body without causing it to break
• How does an object move when a different set of forces is applied
• How does motion change with time
• What happens when bodies collide, an example of impact

All engineers require a basic understanding of mechanics, whatever their precise discipline. That is why it forms part of the core material.

▣  MECHANICS – the study of motion 

• Motion can be either translation or deflection

▣  Motion needs Energy

▣  Mechanics

• the study of Energy
• the study of how energy is converted from one form to another

▣  Potential Energy

• The ability (potential) to do work

Many forms

▣  Mechanical

• Gravitational Potential Energy =PE
• Motion (kinetic energy) = KE

▣  Electrical

▣  Chemical

Where does it come from?

▣  The sun

The Universal Law of Motion & Position

"Principle of Least Action"

“When a change occurs in nature, the quantity of action necessary for the change is the least possible”

(M. de Maupertuis Lyon 1756)

“People were using the Principle of Least Action before they knew what it was”

(Wittgenstein: Tractus Philosophicus Logicus)

Why Light travels in straight lines, Laws of reflection and Refraction, Quantum Mechanics, Newton’s Laws, Laws of everything!!

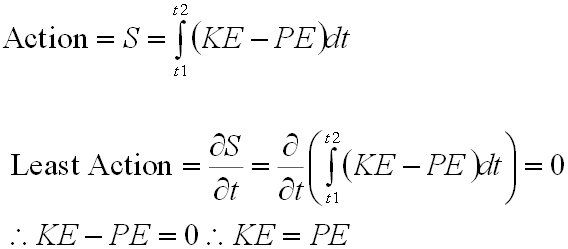
Conservation of Energy

▣  Energy is conserved

• it can neither be created or destroyed
• it can only be transformed from one form to another

Where

UT =  Total Potential Energy

Um =  Mechanical Potential

Energy

Uc =  Chemical Potential

Energy

Ue =  Electrical Potential

Energy

Etc……

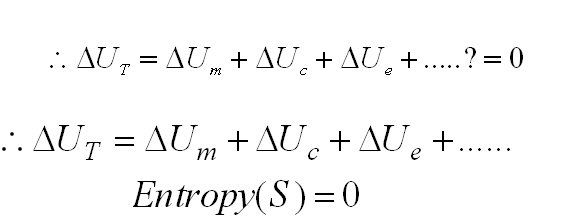
Where

En – energy

Tropy – Trope (form)

Entropy –  energy of change of form